Basics of Light, Lighting Concepts and Color
Light as energy
Light is remarkable. It is something we take for granted every day, but it's not something we stop and think about very often or even try and define. Let's take a few minutes and try and understand some things about light.
Simply stated, light is nature's way of transferring energy through space. We can complicate it by talking about interacting electric and magnetic fields, quantum mechanics, and all of that, but just remember--light is energy.
Light travels very rapidly, but it does have a finite velocity. In vacuum, the speed of light is 186,282 miles per second (or nearly 300,000 kilometers per second), which is really humming along! However, when we start talking about the incredible distances in astronomy, the finite nature of light's velocity becomes readily apparent. It takes about two and a half seconds, for instance, for a radio communication travelling at the speed of light to get to the moon and back.

You might find it interesting to remember, the next time you watch a beautiful sunrise or sunset, that the sun itself actually dipped below the horizon eight minutes earlier--it takes that long for the light to reach the Earth! And, of course, every newspaper article you ever read about astronomy will always include the required statement, "A light year is the distance light travels in one year at the speed of 186,282 miles per second, about 6 trillion miles." (Well, 5.8 trillion miles actually, but what's 200 billion miles among friends?)
We should also highlight right up front that light is more generally referred to as electromagnetic radiation. Okay, we used a big word. It had to happen sooner or later. But too often when we say "light" it is mistaken to mean "optical light," which is roughly the radiation visible to our eyes. Visible light is a tiny portion of a huge smorgasboard of light called the electromagnetic spectrum. For our convenience, we break this smorgasboard up into different courses (appetizer, salad, etc.) and refer to them by name, such as gamma-rays, X-rays, ultraviolet, optical, infrared, and radio. However, it is important to remember that they are all just light. There are no "breaks" and no hard boundaries in the electromagnetic spectrum--just a continuous range of energy.
Particles and Waves
Physics experiments over the past hundred years or so have demonstrated that light has a dual nature. In many instances, it is convenient to represent light as a "particle" phenomenon, thinking of light as discrete "packets" of energy that we call photons. Now in this way of thinking, not all photons are created equal, at least in terms of how much energy they contain. Each photon of X-ray light contains a lot of energy in comparison with, say, an optical or radio photon. It is this "energy content per photon" that is one of the distinguishing characteristics of the different ranges of light described above. Even though it is not strictly correct, it is hard not to think of a beam of light as a collection of little "light bullets" all strung together in a row.

The "wave" model of light.
The other way of representing light is as a wave phenomenon. This is somewhat more difficult for most people to understand, but perhaps an analogy with sound waves will be useful. When you play a high note and a low note on the piano, they both produce sound, but the main thing that is different between the two notes is the frequency of the vibrating string producing the sound waves--the faster the vibration the higher the pitch of the note. If we now shift our focus to the sound waves themselves instead of the vibrating string, we would find that the higher pitched notes have shorter wavelengths, or distances between each successive wave. Likewise (and restricting ourselves to optical light for the moment), blue light and red light are both just light, but the blue light has a higher frequency of vibration (or a shorter wavelength) than the red light.
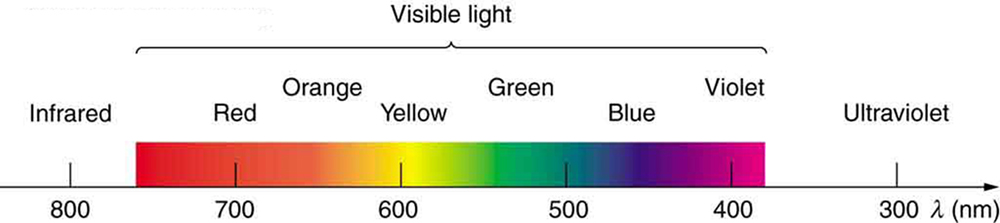
The colors of the familiar "rainbow" of visible light correspond to differing wavelengths of the light, here shown on a nanometer scale. The wavelengths get successively larger as one moves from left to right. Optical light runs from about 400 to 700 nanometers.
It's the same way as we move throughout the electromagnetic spectrum. Each range of light we have defined above corresponds to a range of frequencies (or wavelengths) of light vibrations. These wavelengths are one of the primary indicators we use to describe light and spectra on a graph. Displaying a spectrum as a graph instead of just a color bar allows us to measure the light.
For instance, the "rainbow" of color shown in the figure above is what you see when you pass white light through a prism. What may not be obvious, however, is that the "intensity" or brightness of the light is also changing along with the colors. If we converted the "rainbow" into a graph of light intensity versus wavelength, it would look like this:
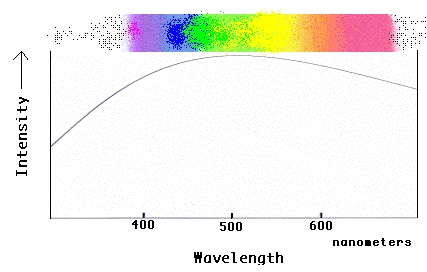
The familiar "rainbow" of the visible spectrum can be converted into a graph that shows how the intensity of the light changes along the spectrum.
Notice that the spectrum is brightest in the middle (yellow-green region) and drops off in both directions (toward red and blue). This was not obvious from the rainbow version of the spectrum! Also notice that the "intensity" of the light in the graph does not stop at the "ends" of the rainbow spectrum that is visible to our eyes! The light continues beyond what we can see in both directions, which we can see in the graph but not by looking at the rainbow. Astronomers use graphical spectra most of the time because they can get more information out of the light this way, and because they can still plot and analyze light that is not directly visible to our eyes!
Now we mentioned that the energy of each photon of light was also a basic property. It turns out that there is a simple relationship between the energy of a photon and the corresponding wavelength of that photon:
E(photon) = (constant) / (wavelength)
This simple equation basically ties together the particle and wave nature of light by permitting us to convert back and forth from wavelengths to photons and photons to their corresponding wavelengths. This equation is also in accord with what we said earlier...an X-ray photon has a large energy (and a small wavelength) compared with a photon of optical light.
Interaction of Light with Matter: Absorption and Emission of Light
It should come as no surprise to you that atoms and molecules (which are simply bound collections of two or more atoms) can absorb light (= energy!). If they didn't, you could simply flick a light on and off, and then sit back while the photons continued to bounce around the room! Likewise, infrared light (= heat = energy!) wouldn't do any good in heating up your home in the winter if it didn't get absorbed by matter. Higher energy light photons, like X-rays, tend to want to plow through more matter before they get absorbed. (Hence, their use in medical imaging: they can pass through your "soft" tissue, but are more readily absorbed in your bones, which are denser.) How and why do photons get absorbed by matter?
Well, it's time to develop another conceptual device to help us understand this process. In physics, we often find it helpful to pretend we are looking at a single atom. Atoms are made up of protons, neutrons, and electrons, and each chemical element has a specific number of them--that's what makes them different! Protons (and neutrons) are more massive than electrons, and so we sometimes visualize an atom as a miniature solar system, with the heavy particles at the center (the nucleus) and the electrons whizzing around in specific "orbits" like planets. (In reality, this picture is not very accurate. Electrons are not thought to be little balls "in orbit" around a nuclear "sun." However, if you get the idea that the electrons are only found at specific, discrete "distances" from the nucleus, and that each allowed distance corresponds to a different "energy level" for the electron, that would be closer to reality.)
Without delving into atomic physics and quantum mechanics too far, let us just take the following statement for granted for now: the electrons bound to any particular atom can only be found in certain, specific energy levels with respect to the atom's nucleus. The hydrogen atom only contains one proton and one electron, and is the simplest (and most common) element in the Universe, so let's use it as an example. The figure [TBD] shows a schematic hydrogen atom where instead of drawing the allowed "orbits" for the electron we draw vertically-displaced lines to represent the allowed energy levels for the electron.
If left undisturbed, our hydrogen atom likes to bind its electron as tightly as it can, and so we would find the electron in the lowest energy level, which is called the "ground state." However, if our atom is immersed in a beam of light from, say, a nearby star, sooner or later the atom will encounter a photon with an energy that is just the right amount to jump the electron up to the next higher energy level. Voila! The photon gets absorbed, and is "gone" from the beam of light coming from the star! Since the absorbed photon had a specific energy, this absorption occurs at a specific wavelength in the spectrum.
Now our hydrogen atom is in what is called an "excited" state, sort of like a kid right before Halloween. However, as all parents know, this is not the natural state of a child, and it's not the natural state of an atom either. If no other photons are absorbed by the atom, the electron will eventually drop back down to the lower energy ground state. However, the atom has to lose energy to do this, and so it releases a photon of the same energy as the one it absorbed (albeit most likely into some other direction from which it was absorbed). This process is called emission because a photon of light is emitted by the atom, again at a very specific wavelength.
Of course, the atom could have absorbed another photon with just the right energy to jump up another energy level, or even two or three or more. Likewise, after each of these possible excitations of the atom, the electron could jump back down one or more steps, emitting photons as it went. If a photon with a sufficiently large energy gets absorbed, it can even cause an electron to become unbound from its nucleus, a process that is called ionization. Our crippled hydrogen atom could then no longer absorb or emit light until it manages to capture a free electron back into a bound energy level.
We have been discussing one specific transition or "energy jump" in one atom, but of course in any physical system there are many atoms. In a hydrogen gas, all of the separate atoms could be absorbing and emitting photons corresponding to the whole group of "allowed" transitions between the various energy levels, each of which would absorb or emit at the specific wavelengths corresponding to the energy differences between the energy levels. This pattern of absorptions (or emissions) is unique to hydrogen--no other element can have the same pattern--and causes a recognizable pattern of absorption (or emission) lines in a spectrum.
Filter Products
0 item